Interference

From Wikipedia, the free encyclopedia

Jump to: navigation, search

For other uses, see Interference (disambiguation).

Interference of two circular waves - Wavelength (decreasing bottom to top) and Wave centers distance (increasing to the right). Absolute value snapshots of the (real-valued, scalar) wave field. As time progresses, the wave fronts would move outwards from the two centers, but the dark regions (destructive interference) stay fixed.

Interference is the addition (superposition) of two or more waves that results in a new wave pattern.

As most commonly used, the term interference usually refers to the interaction of waves which are correlated or coherent with each other, either because they come from the same source or because they have the same or nearly the same frequency.

Two non-monochromatic waves are only fully coherent with each other if they both have exactly the same range of wavelengths and the same phase differences at each of the constituent wavelengths.

The total phase difference is derived from the sum of both the path difference and the initial phase difference (if the waves are generated from 2 or more different sources). It can then be concluded whether the waves reaching a point are in phase(constructive interference) or out of phase (destructive interference).

1 Theory
2 Experiments
3 Interference patterns
4 Constructive and destructive interference
5 General Quantum Interference
6 Examples
7 See also
8 References
9 External links

[edit] Theory

The principle of superposition of waves states that the resultant displacement at a point is equal to the sum of the displacements of different waves at that point. If a crest of a wave meets a crest of another wave at the same point then the crests interfere constructively and the resultant wave amplitude is greater. If a crest of a wave meets a trough of another wave then they interfere destructively, and the overall amplitude is decreased.

This form of interference can occur whenever a wave can propagate from a source to a destination by two or more paths of different length. Two or more sources can only be used to produce interference when there is a fixed phase relation between them, but in this case the interference generated is the same as with a single source; see Huygens' principle.

[edit] Experiments

Thomas Young's double-slit experiment showed interference phenomena where two beams of light which are coherent interfere to produce a pattern.

The beams of light both have the same wavelength range and at the center of the interference pattern. They have the same phases at each wavelength, as they both come from the same source.

[edit] Interference patterns

For two coherent sources, the spacial separation between sources is half the wavelength times the number of nodal lines

Light from any source can be used to obtain interference patterns, for example, Newton's rings can be produced with sunlight. However, in general white light is less suited for producing clear interference patterns, as it is a mix of a full spectrum of colours, that each have different spacing of the interference fringes. Sodium light is close to monochromatic and is thus more suitable for producing interference patterns. The most suitable is laser light because it is almost perfectly monochromatic.

[edit] Constructive and destructive interference

Interference pattern produced with a Michelson interferometer. Bright bands are the result of constructive interference while the dark bands are the result of destructive interference.

Consider two waves that are in phase,with amplitudes $A 1$ and $A 2$ . Their troughs and peaks line up and the resultant wave will have amplitude $A = A 1 + A 2$ . This is known as constructive interference.

If the two waves are pi radians, or 180°, out of phase, then one wave's crests will coincide with another wave's troughs and so will tend to cancel out. The resultant amplitude is $A = | A 1 - A 2 |$ . If $A 1 = A 2$ , the resultant amplitude will be zero. This is known as destructive interference.

When two sinusoidal waves superimpose, the resulting waveform depends on the frequency (or wavelength) amplitude and relative phase of the two waves. If the two waves have the same amplitude $A$ and wavelength the resultant waveform will have an amplitude between 0 and $2 A$ depending on whether the two waves are in phase or out of phase.

combined waveform
wave 1
wave 2
	Two waves in phase	Two waves 180° out of phase

[edit] General Quantum Interference

Two point interference in a ripple tank.

If a system is in state $ψ$ its wavefunction is described in Dirac or bra-ket notation as:

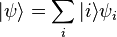
$|\psi \rang = \sum_i |i\rang \psi_i$

where the $|i\rang$ s specify the different quantum "alternatives" available (technically, they form an eigenvector basis) and the $ψ i$ are the probability amplitude coefficients, which are complex numbers.

The probability of observing the system making a transition or quantum leap from state $ψ$ to a new state $φ$ is the square of the modulus of the scalar or inner product of the two states:

$prob(\psi \Rightarrow \phi) = |\lang \psi |\phi \rang|^2 = |\sum_i\psi^*_i \phi_i |^2 = \sum_{ij} \psi^*_i \psi_j \phi^*_j\phi_i= \sum_{i} |\psi_i|^2|\phi_i|^2 + \sum_{ij;i \ne j} \psi^*_i \psi_j \phi^*_j\phi_i$

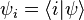where $\psi_i = \lang i|\psi \rang$ (as defined above) and similarly 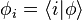$\phi_i = \lang i|\phi \rang$ are the coefficients of the final state of the system. * is the complex conjugate so that $\psi_i^* = \lang \psi|i \rang$ etc.

Now let's consider the situation classically and imagine that the system transited from 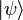$|\psi \rang$ to $|\phi \rang$ via an intermediate state $|i\rang$ . Then we would classically expect the probability of the two-step transition to be the sum of all the possible intermediate steps. So we would have

The classical and quantum derivations for the transition probability differ by the presence, in the quantum case, of the extra terms 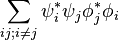$\sum_{ij;i \ne j} \psi^*_i \psi_j \phi^*_j\phi_i$ ; these extra quantum terms represent interference between the different 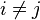$i \ne j$ intermediate "alternatives". These are consequently known as the quantum interference terms, or cross terms. This is a purely quantum effect and is a consequence of the non-additivity of the probabilities of quantum alternatives.

The interference terms vanish, via the mechanism of quantum decoherence, if the intermediate state $|i\rang$ is measured or coupled with the environment^[1]^[2].

[edit] Examples

A conceptually simple case of interference is a small (compared to wavelength) source - say, a small array of regularly spaced small sources (see diffraction grating).

Consider the case of a flat boundary (say, between two media with different or simply a flat mirror, onto which the plane wave is incident at some angle. In this case of continuous distribution of sources, constructive interference will only be in specular direction - the direction at which angle with the normal is exactly the same as the angle of incidence. Thus, this results in the law of reflection which is simply the result of constructive interference of a plane wave on a plane surface.