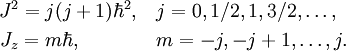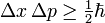Planck constant
From Wikipedia, the free encyclopedia
The Planck constant (denoted h) is a physical constant that is used to describe the sizes of quanta. It plays a central role in the theory of quantum mechanics, and is named after Max Planck, one of the founders of quantum theory. A closely-related quantity is the reduced Planck constant (also known as Dirac's constant and denoted ħ, pronounced "h-bar"). The Planck constant is also used in measuring energy emitted as photons, such as in the equation E=hν, where E is energy, h is Planck's constant, and ν (Greek letter nu) is frequency.
The Planck constant and the reduced Planck constant are used to describe quantization, a phenomenon occurring in subatomic particles such as electrons and photons in which certain physical properties occur in fixed amounts rather than assuming a continuous range of possible values.
Contents
|
[edit] Significance of the size of Planck's constant
Expressed in the SI units of joule seconds (J·s), the Planck constant is one of the smallest constants used in physics. The significance of this is that it reflects the extremely small scales at which quantum mechanical effects are observed, and hence why we are not familiar with quantum physics in our everyday lives in the way that we are with classical physics. Indeed, classical physics can essentially be defined as the limit of quantum mechanics as the Planck constant tends to zero.
In natural units, the Dirac constant is taken as 1 (i.e., the Planck constant is 2·π), as is convenient for describing physics at the atomic scale dominated by quantum effects.
[edit] Units, value and symbols
The Planck constant has dimensions of energy multiplied by time, which are also the dimensions of action. In SI units, the Planck constant is expressed in joule seconds (J·s). The dimensions may also be written as momentum times distance (N·m·s), which are also the dimensions of angular momentum. Often the unit of choice is eV·s, because of the small energies that are often encountered in quantum physics.
The value of the Planck constant is:
The two digits between the parentheses denote the standard uncertainty in the last two digits of the value.
The value of the Dirac constant is:
The figures cited here are the 2006 CODATA-recommended values for the constants and their uncertainties. The 2006 CODATA results were made available in March 2007 and represent the best-known, internationally-accepted values for these constants, based on all data available as of 31 December 2006. New CODATA figures are scheduled to be published approximately every four years.
Unicode reserves codepoints U+210E (ℎ) for the Planck constant, and U+210F (ℏ) for the Dirac constant.
[edit] Origins of Planck's constant
The Planck constant,  , was proposed in reference to the problem of black-body radiation. The underlying assumption to Planck's law of black body radiation was that the electromagnetic radiation emitted by a black body could be modeled as a set of harmonic oscillators with quantized energy of the form:
, was proposed in reference to the problem of black-body radiation. The underlying assumption to Planck's law of black body radiation was that the electromagnetic radiation emitted by a black body could be modeled as a set of harmonic oscillators with quantized energy of the form:
 is the quantized energy of the photons of radiation having frequency (Hz) of
is the quantized energy of the photons of radiation having frequency (Hz) of  (nu) or angular frequency (rad/s) of
(nu) or angular frequency (rad/s) of  (omega).
(omega).
This model proved extremely accurate, but it provided an intellectual stumbling block for theoreticians who did not understand where the quantization of energy arose — Planck himself only considered it "a purely formal assumption". This line of questioning helped lead to the formation of quantum mechanics.
In addition to some assumptions underlying the interpretation of certain values in the quantum mechanical formulation, one of the fundamental corner-stones to the entire theory lies in the commutator relationship between the position operator  and the momentum operator
and the momentum operator  :
:
where  is the Kronecker delta. For more information, see the mathematical formulation of quantum mechanics.
is the Kronecker delta. For more information, see the mathematical formulation of quantum mechanics.
[edit] Usage
The Planck constant is used to describe quantization. For instance, the energy (E) carried by a beam of light with constant frequency (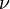 ) can only take on the values
) can only take on the values
It is sometimes more convenient to use the angular frequency  , which gives
, which gives
Many such "quantization conditions" exist. A particularly interesting condition governs the quantization of angular momentum. Let J be the total angular momentum of a system with rotational invariance, and Jz the angular momentum measured along any given direction. These quantities can only take on the values
Thus,  may be said to be the "quantum of angular momentum".
may be said to be the "quantum of angular momentum".
The Planck constant also occurs in statements of Heisenberg's uncertainty principle. Given a large number of particles prepared in the same state, the uncertainty in their position, Δx, and the uncertainty in their momentum (in the same direction), Δp, obey
where the uncertainty is given as the standard deviation of the measured value from its expected value.
There are a number of other such pairs of physically measurable values which obey a similar rule.
[edit] Dirac constant
The Dirac constant or the "reduced Planck constant",  , differs only from the Planck constant by a factor of 2π. The Planck constant is stated in SI units of measurement, joules per hertz, or joules per (cycle per second), while the Dirac constant is the same value stated in joules per (radian per second).
, differs only from the Planck constant by a factor of 2π. The Planck constant is stated in SI units of measurement, joules per hertz, or joules per (cycle per second), while the Dirac constant is the same value stated in joules per (radian per second).
In essence, the Dirac constant is a conversion factor between phase (in radians) and action (in joule-seconds) as seen in the Schrödinger equation. The Planck constant is similarly a conversion factor between phase (in cycles) and action. All other uses of Planck's constant and Dirac's constant follow from that.



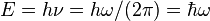
![[\hat{p_i}, \hat{x_j}] = -i \hbar \delta_{ij}](http://upload.wikimedia.org/math/a/0/5/a05601a21cbab6cd64e2d6c4ffc842cb.png)