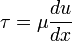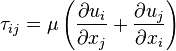Newtonian fluid

From Wikipedia, the free encyclopedia

Jump to: navigation, search

Continuum mechanics

Key topics
Conservation of mass Conservation of momentum Navier-Stokes equations
Classical mechanics
Stress · Strain · Tensor
Solid mechanics
Solids · Elasticity Plasticity · Hooke's law Rheology · Viscoelasticity
Fluid mechanics
Fluids · Fluid statics Fluid dynamics · Viscosity · Newtonian fluids Non-Newtonian fluids Surface tension
Scientists
Newton · Stokes · others
This box: view • talk • edit

A Newtonian fluid (named for Isaac Newton) is a fluid that flows like water—its stress versus rate of strain curve is linear and passes through the origin. The constant of proportionality is known as the viscosity.

A simple equation to describe Newtonian fluid behaviour is

$\tau=\mu\frac{du}{dx}$

where

τ

is the shear stress exerted by the fluid ("drag") [Pa]

μ

is the fluid viscosity - a constant of proportionality [Pa·s]

$\frac{du}{dx}$ is the velocity gradient perpendicular to the direction of shear [s⁻¹]

In common terms, this means the fluid continues to flow, regardless of the forces acting on it. For example, water is Newtonian, because it continues to exemplify fluid properties no matter how fast it is stirred or mixed. Contrast this with a non-Newtonian fluid, in which stirring can leave a "hole" behind (that gradually fills up over time - this behaviour is seen in materials such as pudding, oobleck, or, to a less rigorous extent, sand), or cause the fluid to become thinner, the drop in viscosity causing it to flow more (this is seen in non-drip paints, which brush on easily but become more viscous when on walls).

For a Newtonian fluid, the viscosity, by definition, depends only on temperature and pressure (and also the chemical composition of the fluid if the fluid is not a pure substance), not on the forces acting upon it.

If the fluid is incompressible and viscosity is constant across the fluid, the equation governing the shear stress, in the Cartesian coordinate system, is

$\tau_{ij}=\mu\left(\frac{\partial u_i}{\partial x_j}+\frac{\partial u_j}{\partial x_i} \right)$

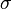with comoving stress tensor $\mathbb{P}$ (also written as $\mathbf{\sigma}$ )

$\mathbb{P}_{ij}= - p \delta_{ij} + \mu\left(\frac{\partial u_i}{\partial x_j}+\frac{\partial u_j}{\partial x_i} \right)$

where, by the convention of tensor notation,

τ i j

is the shear stress on the

i t h

face of a fluid element in the

j t h

direction

u i

is the velocity in the

i t h

direction

x j

is the

j t h

direction coordinate

If a fluid does not obey this relation, it is termed a non-Newtonian fluid, of which there are several types.

v • d • e General subfields within physics
Classical mechanics · Electromagnetism · Thermodynamics · Statistical mechanics · Quantum mechanics · Relativity · High energy physics · Condensed matter physics · Atomic, molecular, and optical physics

Retrieved from "http://en.wikipedia.org/wiki/Newtonian_fluid"

Categories: Continuum mechanics | Fluid dynamics